One of the most striking  features of the domestic dog is its enormous variety of size and shape, not found in any other species, either wild or domestic one. The cardigan corgi is an  example of a short-legged, long bodied (chondrodysplastic)  breed.    Research suggest that a single mutation appeared at very early stage in domestic dogs development, and it is commonly found in small breeds.

Parker et al. (2009), using multibreed association analysis in domestic dogs, demonstrate that expression of a recently acquired retrogene encoding fibroblast growth factor 4 (fgf4) is strongly associated with chondrodysplasia, a short-legged phenotype that defines at least 19 dog breeds, including dachshund, corgi and basset hound. 

Size, together with many other features, that contribute to dog appearance, is a polygenic, quantitative trait . This means that it is measureable (e.g. size, or height at withers in cms, body weight in kgs), and variable within the breed.  These traits depend mostly on additive influence of many (the exact number is not known) different genes, and  are also influenced by environment. In case of size, the final result depends both on individual genetic makeup and proper rearing.

Recent, more advanced studies, employing sophisticated methods of molecular genetics, identified  6 loci of quantitative genes (QTLs) that influence size and shape in dogs (Chase et al.2002).    Mutations within  IGF-1 (insulin-like growth factor 1) were found  of crucial importance during  puppy growth; they also influence metabolism in adult dogs (Sutter et al. 2007) . It was also shown  that small and miniature breeds are usually homozygous in three IGF-1 haplotypes.

Specimens of any breed vary in size, and this variation is usually included in their respective breed standards. In case of cardigan welsh corgi the standard reads:  “ideal height at withers 30 cms”. However, in any group of cardis there will be probably no two dogs of exactly same size.  Where does this variation come from (apart from different rearing conditions)? To begin, assume that all cardigans have same genes, encoding the actual size, and their accumulated action enable any given specimen to grow to 30 cms. The only difference is in two pairs of additive alleles, A1A2 and B1B2. Assume also that A1 and B1 add 1 cm to  the actual height, whereas A2 and B2 do not increase the size. When two specimen of identical  heterozygous genotypes  are mated together,  there will be 16 possible combinations of gametes (see the table) :

TABLE 1:  


Parents :  A1A2B1B2 32 cm x A1A2B1B2 32 cm  

In resulting progeny there may be:

      1/16 puppies of 34 cms height  -   6.25%
   4/16 puppies of 33 cms height  -  25%
     6/16 puppies of 32 cms height  -  37.5%
  4/16 puppies of 31 cms height  -  25%
      1/16 puppies of 30 cms height  -    6.25%

The majority of puppies will be these of medium height (same as their parents); however, there will be also several specimens distinctly smaller  or  bigger than parents.

It is important to realize that the above example is most probably simplified. Variation in size results not only from additive action of genes. In some cases there might be also two other types of co-operation within or  between alleles involved. Assume that in the given pair of alleles  - A1A2 -  A1 is completely dominant to A2. In this case a dog of A1A1 genotype does not differ in size to a dog of A1A2 (in our example this will give at least 32 cms, the rest depending on locus B). Yet the first dog (A1A1) would pass the “big size” gene to all its puppies, whereas  the other dog (A1A2) – only to half of them.

Additionally,  genes of one allele pair may be epistatic to genes of the  other allele. An epistatic gene (or its pair)  blocks other gene; in our example it may happen that A2A2 pair block completely the action of B1 gene… Then a dog of A2A2B1B1 genotype is  only 30 cms tall, and not 32 cms. As far as size is concerned, it is possible that the presence of certain mutated  IGF 1 allele, which results in low production of insulin,  leads itself to small size, even though the combination of other genes could bring bigger size. Epistatic co-operation between different genes is not hereditary: A2A2B1B1 dog will pass B1 and A2 alleles to its puppies;  if they get A1 allele from the second parent, epistatic effect will not appear. 

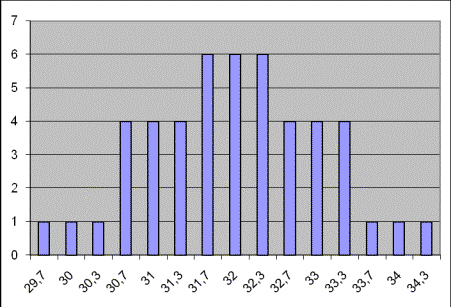
To make the matter even more complicated, quantitative traits are – as written earlier -   influenced by environment. This influence (optimal  or less favorable conditions during growth period) may result in increase or decrease of the actual height, let say by  +0.3 to -0.3 cm. Puppies, which are genetically 34 cms, will show slightly different size – from 33.7 up to 34.3 cms, depending on whether they have been reared in excellent, decent, or poor conditions.

Graph 1 shows size variation in any given group of cardigan welsh corgis:

 Graph 1

As it is seen on the graph, size variation varies from 29.7 to 34.3 cms,  and the majority of dogs remains within medium size range. As the number of genes, contributing to size, is unknown, it is worth remembering that the number of possible genotypes may be enormous. In case of only one pair of alleles there are 3 possible genotypes, in case of two pairs – 9 different genotypes, in case of n  pairs -  3ⁿ  different genotypes. Just as few as 6 pairs of alleles result  in 3⁶ = 729 different genotypes possible. 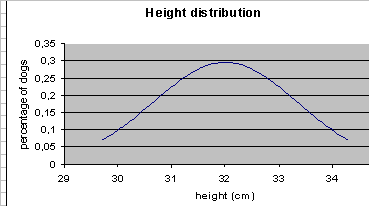Considering  additional co-operation of genes, as described earlier, and environmental influences, it does not come as a big surprise that the number of possible phenotypes is almost endless, and they might be very close to each other.    They cannot be shown on the graph like Graph 1,  for they form continuum, bordered by two values – the highest and the lowest one, as shown on Graph 2.

GRAPH 2.  

Typically, variability of any quantitative trait can be graphically presented in the form of bell- shaped curve (Gauss curve) with its peak at mean value. This type of distribution is known as “normal”. It can be seen that the majority of dogs, i.e. 1/3 of the total population, are these of medium size – 32 cms.  Size of appr. 68% of all dogs remains within standard deviation (SD) range from the mean value (in our case – 32 cms), and 98% - within double SD range. This is  only an example – the actual dispersion, or variability in the population can be bigger or smaller, resulting in more flat or more steep  curve.

In  breeding farm animals selection may be  aimed at increasing size and weight (e. g. poultry, swine). In pure bred dogs, with just a few exceptions,  such type of selection does not take place, at least consciously, and probably no one has ever selected cardigans for  their size only. Nevertheless, they definitely  have become bigger,  and one may ask the reason.

There may be at least three reasons that lead to increase in cardigan size:

1.      Improved environmental conditions, mainly balanced feeding, that enables a dog to fully develop  its genetic potentials.

2.      Unconscious selection. Very few breed standards call for eliminating from breeding any specimen above or under specified height. In Shetland Sheepdogs breeders avoid using dogs that are obviously oversized, as height of more than +2.5 cms above the standard  is considered a serious fault (at least in GB and Europe).  No cardigan breeder will probably avoid breeding oversized animals; moreover, in practice such specimens are favored in conformation rings as they look showy. Contrary, a small specimen, especially a male, will be probably faulted (or at least ignored by judges) and thus not used, or seldom used in breeding. In our simplified example (Table 1) an  A2A2B2B2 male will be the one which is not used, whereas A1A1B1B1 will be probably  used frequently.  By culling smallest specimens the mean height of dogs in subsequent generation will be slightly bigger than that of parental generation.   In our example, if  all dogs which are smaller than 30 cms are not used in breeding, the mean height of parental generation will increase to 32.3 cms. Such “unconscious” selection has low intensity (especially considering that size itself is seldom the main factor in selection) , yet it accumulates in subsequent generations and is growing slowly, but  steadily, and the mean height of the population gradually  increases.

(N.B:  The actual increase depends also on  heritability (h²⁾  of the trait. This is one of the most important parameters used  in scientific  breeding,  and indicates what  part of the total variability in phenotypes is  inherited.  h² of  any given trait can vary from 0 to 1, or 100%  – the higher it is, the bigger is genetic  compound of the trait).

3.      It is commonly known that small dogs produce smaller litters than dogs of large breeds (Willis, 1989). Also in one breed, e.g. Yorkshire Terrier, small bitches deliver small litters. Thus small cardigan bitches may produce smaller litters and introduce  fewer puppies  to the next generation than  bigger bitches. In the next generation there will be more specimens of bigger size and carrying such genotype. 

   Miroslaw Redlicki

 (revised by Katarzyna Fiszdon, PhD, Dept. Of Animal Breeding, Warsaw University of Life Sciences)

Literature:

Parker H.G. et al.  An Expressed fgf4 Retrogene Is Associated with Breed-Defining Chondrodysplasia in Domestic Dogs, Science: Vol. 325 (2009), pp. 995 – 998

  Chase K. et al. Genetic basis for systems of skeletal quantitative traits: principal component analysis of the canid skeleton, Proc. Natl. Acad.  Sci. U.S.A. Volume: 99 (2002) pp. 9930-9935

  Sutter N.B. et al.  A single ancient IGF-1 allele causes small size in dogs. Science, Vol. 316, (2007), pp. 112-115

  Willis M.B. Genetics of the Dog, 1989, Howell Book House